Quote of the Day
Books are standing counselors and preachers, always at hand, and always disinterested; having this advantage over oral instructors, that they are ready to repeat their lesson as often as we please.
Introduction
Figure 1: Strokkur Geyser in Iceland. Geysers are powered by the Earth's internal heat. The Earth remains hot because of radioactivity and the residual heat of formation. (Source)
Everyday I visit the site refdesk.com because it contains little facts and figures that someone like me really gets into. A few days ago, the following piece of trivia was in their "Fact of the Day" section [Source-item #5]:
Holes drilled as deep as 5 miles into the Earth's surface reveal that the rock temperature increases about 37 °F per 320 feet.
You usually see this number stated as 20 °C/km (example). This piece of trivia caught my eye because it is the Earth's temperature gradient near the surface. If it is relatively constant around the world, we should be able to use this number to compute the total heat output of the Earth. I did a bit of research, and I quickly discovered that there are many nuances to working with geophysics. I also learned that geophysics is a really interesting field with a lot of great mathematics. Unfortunately, it is a field where direct measurements of significant Earth features, like the core, cannot be obtained. It may be the case that Earth is a billion years old, but very little is actually known about such an important bit of it as the core. It is difficult to obtain even basic properties of materials (like the density of iron) at the temperatures and pressures that exist at the Earth's core.
Even with these difficulties, I thought I could do a bit of math to come up with rough approximations for some of the numbers I encountered in my research. Let's look at two numbers:
- PTotal = 44 to 47 TerraWatts (TW): The total heat output of the Earth.
The Earth has multiple sources for its internal heat: radioactive decay (see this blog post), the initial heat present since the formation of the Earth, latent heat from the solidification of the core, tidal heating, etc. If we know that temperature gradient near the Earth's surface, we should be able to estimate the total amount of heat being generated from these sources within the Earth.
- Solidification rate = 1 mm/year: The rate at which the inner core is solidifying.
The Earth is slowly cooling. One result of this cooling is that the solid inner core is slowly growing.
One concept that intrigues me is the idea that the Earth would be warm underground even without the Sun present. See the Wikipedia for an interesting discussion of this topic. My favorite science fiction story is After Worlds Collide, which is tale that includes a rogue planet called Bronson Beta. This rogue planet survived a very long trip through the bitter cold of interstellar space. Its former inhabitants had built deep underground tunnels that provided a warm sanctuary for travelers from Earth.
Background
Structure of the Earth
Figure 2 shows the layered structured of the Earth's interior (Source).
Figure 2: Layered Structure of the Earth.
We can only take direct measurements of the rocks down to about 12 km. In fact, we have never drilled deep enough to reach the mantle, though we are trying. We can measure things like temperature gradients near the surface and we can also analyze the rumblings from within the Earth to determine the basic structure of the layers.
Analysis
Power Flow and Temperature Gradient
Equation 1 relates power flow to the temperature gradient and the crust's thermal conductivity.
| Eq. 1 |  |
where
- q is the heat flow density [mW/m2]
- ? is the thermal conductivity of the material being measured [W/(m K)]
is the temperature gradient [K/m] with respect to depth (d)
There are a number of difficulties in applying Equation 1 over the Earth's surface. A major one is the wide variation in the thermal conductivity of the crust. Generally, we work with averages. Figure 3 shows a graph of the thermal conductivity of crustal rocks versus temperature (Source).
Figure 3: Thermal Conductivity of the Crust as a Function of Temperature.
The Wikipedia uses a value of 3.0 W/(m K) for the thermal conductivity of the continental granite. They use this value to estimate the heat flow from a square meter of continent as shown in Equation 2.
| Eq. 2 | ![\displaystyle {{q}_{Continent}}\approx 3.0\left[ \frac{\text{W}}{\text{m}\cdot \text{K}} \right]\cdot 20\left[ \frac{20\text{K}}{1000\text{ m}} \right]=60\frac{\text{mW}}{{{\text{m}}^{2}}}](https://s0.wp.com/latex.php?latex=%5Cdisplaystyle+%7B%7Bq%7D_%7BContinent%7D%7D%5Capprox+3.0%5Cleft%5B+%5Cfrac%7B%5Ctext%7BW%7D%7D%7B%5Ctext%7Bm%7D%5Ccdot+%5Ctext%7BK%7D%7D+%5Cright%5D%5Ccdot+20%5Cleft%5B+%5Cfrac%7B20%5Ctext%7BK%7D%7D%7B1000%5Ctext%7B+m%7D%7D+%5Cright%5D%3D60%5Cfrac%7B%5Ctext%7BmW%7D%7D%7B%7B%7B%5Ctext%7Bm%7D%7D%5E%7B2%7D%7D%7D&bg=ffffff&fg=000&s=2&c=20201002) |
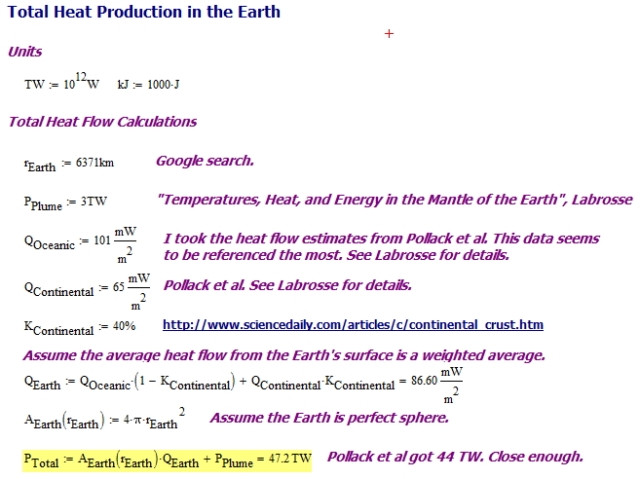
This is a rough calculation. Much more precise measurements and calculations have been performed for the continents and the ocean. Table 1 contains a summary of the power density results and the total geothermal power estimates of different researchers. My work here will use the results from Pollack et al (highlighted) because that is what I find most frequently used in popular discussions.
| Study | Year | Continental (mW-m-2) | Oceanic (mW-m-2) | Total (TW) |
|---|---|---|---|---|
| Williams and von Herzen | 1974 | 61 | 93 | 43 |
| Davies | 1980 | 55 | 95 | 41 |
| Sclater et al. | 1980 | 57 | 99 | 42 |
| Pollack et al. | 1993 | 65 | 101 | 44 |
| Labrosse | 2007 | 65 | 94 | 46 |
Total Power Output
Given the average power density from the continents and the ocean, we can estimate the total power generated within the Earth. This does require that we determine the percentages of continental crust and oceanic crust. For my estimates, I assumed that 40% of the Earth's surface is continental crust (Source). Figure 4 shows my calculations, which includes adding 3 TW for the power from mantle plumes. I saw that some authors made this assumption to model places on the Earth's surface like Yellowstone, which have much higher than average heat flux.
Figure 4: Calculation of the Total Heat Production from The Earth.
My result of 47 TW is a bit higher than those reported in Table 1, but close enough for my rough work here.
Core Solidification Rate
I saw the 1 mm/year solidification rate quoted from a number of sources. Here is a quote from one of these sources.
Over billions of years, Earth has cooled from the inside out causing the molten iron core to partly freeze and solidify. The inner core has subsequently been growing at the rate of around 1 mm year as iron crystals freeze and form a solid mass.
Note that I also saw estimates like 0.3 mm/year and 1 cm/year stated, but rarely so that I will ignore them here. Figure 5 shows my estimate for the latent heat released when 1 mm/year of iron at the inner core boundary solidifies.
Figure 5: Calculation of the Core Heat Generated for a 1 mm Solidification Rate.
My 4 TW number agrees with this source. There is some debate about this number and you will see different values used by different authors.
Conclusion
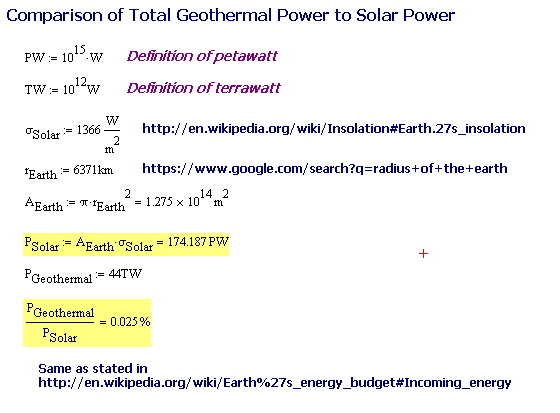
This was an interesting exercise for me. I have always wondered the amount of geothermal energy available on the Earth. The total amount of solar power at the Earth' surface is compared to the total amount of geothermal energy available in Figure 6. There is much more solar power available than geothermal.
Figure 6: Comparison of Total Solar Versus Geothermal Energy Amounts.
Pingback: Sahara Water Math | Math Encounters Blog